Merry Christmas and a Happy New Year to everyone.
Mr.P
Saturday, December 21, 2013
Wednesday, December 18, 2013
Hi Everyone,
I hope everything is going well, and you are learning/getting ready for the exam.
If I get some time I will post couple pictures (perhaps selfies).
Keep up the good work.
Until next type.
From Lousika/Patras, Greece, Europe, World.
Mr. P
I hope everything is going well, and you are learning/getting ready for the exam.
If I get some time I will post couple pictures (perhaps selfies).
Keep up the good work.
Until next type.
From Lousika/Patras, Greece, Europe, World.
Mr. P
Tuesday, December 3, 2013
Trig Help
Hello there Fellow classmates, Irene here. You all probably know me. Im tiny, but hard to miss. Im not going to make this message long and get to the point. I am posting this because I want to help everybody. The last trig test didn't as I planned. :( After that I was determined to get a better grade. I wanted to get more practice, to be more prepared for the next precal test. So I decided to go to the old precal exams and copy every single question that had the word sin, cos, tan, csc, sec, cot, and everything that relates to trig. I started from June 2013 and went all the way back to January of 2007. I wanted to go all the way to 2004, but sadly I got busy with other things and ran out of time. I hope you will make good use of my endless hours of coping and pasting.
PS I didn't get any questions from booklet one of January 2007 because it was malfunctioning :(
Here's the link
https://www.dropbox.com/s/p9x4m3q0ntwey90/trig%20unit.docx
PS I didn't get any questions from booklet one of January 2007 because it was malfunctioning :(
Here's the link
https://www.dropbox.com/s/p9x4m3q0ntwey90/trig%20unit.docx
Hi! Everyone, My name is Shivani. You probably know me, but if you don't I sit right in middle of Harsimran and Ruthanne. Now I shall introduce you with Solving Trigonometric Equations using Identities.
Trignometric Identities are equations involving the trignometric function that are true for every values of the variables involved. Youn can use trignometric identites along with algebric methods to solve trignometric equations.
Example
Find all the solutions of the equation in the interval 2sin2x=2+cosx
The equation contains both sine and cosine functions.
We rewrite the equation so that it contains only cosine functions using the Pythagorean Identity sin2x=1cos2x
Factoring cosx, we get,cosx(2cosx+1)=0
By using zero-product-property,we will get cosx=0,and 2cosx+1=0 whcih yields x= -1⁄2
In the interval [0, 2π), we know that cosx=0 when x=π⁄2 and x= 3π⁄2 .On the other hand, we also know that cosx= -1⁄2 when x= 2π⁄3 and x= 4π⁄3
Therefore, the solutions of the given equation in the interval [0, 2π) are { π⁄2,3π⁄2,2π⁄3,4π⁄3}
I hope you've learned something ! And
Good luck for the test. :)
Trignometric Identities are equations involving the trignometric function that are true for every values of the variables involved. Youn can use trignometric identites along with algebric methods to solve trignometric equations.
Example
Find all the solutions of the equation in the interval 2sin2x=2+cosx
The equation contains both sine and cosine functions.
We rewrite the equation so that it contains only cosine functions using the Pythagorean Identity sin2x=1cos2x
Factoring cosx, we get,cosx(2cosx+1)=0
By using zero-product-property,we will get cosx=0,and 2cosx+1=0 whcih yields x= -1⁄2
In the interval [0, 2π), we know that cosx=0 when x=π⁄2 and x= 3π⁄2 .On the other hand, we also know that cosx= -1⁄2 when x= 2π⁄3 and x= 4π⁄3
Therefore, the solutions of the given equation in the interval [0, 2π) are { π⁄2,3π⁄2,2π⁄3,4π⁄3}
I hope you've learned something ! And
Good luck for the test. :)
Thursday, November 28, 2013
Proving Identities
Hello everybody, my name is Ariana. I am the middle of the 3 ladies who sit at the front table..........probably the one crying (I'm not fully on board and ready to steer this pre-cal ship, so please bear with me on this scribe as I write it while I dangle out, feet soaked in the cold ocean water). If you don't know me by now, feel free to introduce yourself!I I don't bite..... unless necessary :)
Now onto proving identities. Proving an identity is showing how one side of the equation, is the same as the other. (Left Hand Side = Right Hand Side). You can do so by separately simplifying both sides of the identity into identical expressions, Do not attempt to do both at the same time, this process is complicated and prone to much confusion. Also in some cases, you can get both sides identical with only one simplifying one equation. It doesn't matter which side you start with,your best bet is to start with the more complicated one.
As seen in our booklets here are some STRATEGIES (not to be confused with steps) that may help:
- Use known Identities to make substitution ex) tanx=sinx/cosx
- If qaudratics are present, the Pythagorean identity (sin^2theta + cos^2theta=1) or one of its alternate forms can be used. ex) sin^2theta/cos^2theta will become sin^2theta/1-sin^2theta
- Rewrite the expression using only sin and cosine
- Multiply the numerator and the denominator by the conjugate of an expression
- Factor to simplify the expression
Some examples we went over together in class:
Example a)
 |
| I apologize for poor quality pictures |
Starting with the left hand side (since we cant do anything to the right) the first step would be to change everything we can to sine or cosine.
Based on trig identities we know tantheta would become sintheta/costheta, csctheta would become 1/sintheta.
As we can see in the picture the cos and sin would cancel out, leaving us with only 1
Since 1 is what is on the right we have 1=1, LHS=RHS
Example b)
 |
| Quality plus my handwriting gets worse... |
On the LHS, first we change everything to become sine or cosine. Cottheta turns into costheta/sintheta and sec^2 becomes 1/cos^theta.
The costheta on the numerator would cancel with one on the denominator (remember that it's squared, we would still be left with one costheta).
Therefore our LHS is left with 1/sintheta costheta
1/ sintheta costheta is what is on the RHS, LHS=RHS
Example c)
In the LHS we convert tan to become sintheta/costheta.
The next step to this equation is to find the common denominator, which is costheta.
Apply the CD and we get sintheta costheta + sintheta/ cos theta.
Our next instinct might be that the costheta would cancel but that would be wrong because of that addition symbol.
The next step would be to factor out sintheta
Leaving us with LHS: sintheta(costheta+1/costheta)
For the RHS, of course we change to become sin or cosine.
1=sectheta/csctheta becomes *look on pic, for it does not look pretty typed*
Find the common denominator and then I'm pretty sure you already know the steps to simplify further.
The RHS is (costheta+1)sintheta/costheta
LHS=RHS
Example d)
In this special case we start by multiplying by the conjugate.
According to the Pythagorean identity we can change 1-cos^2 into sin^6.
Need I tell you what to do next?
LHS=RHS
 |
Tuesday, November 26, 2013
Trigonometric Identities
GHKNJH-
After wrestling with Blogger for an hour I finally figured out where my invitation email went. So uh, hi guys, I don't want to do this but I'm being forced, I guess. This is Casey, that quiet girl in the back of the classroom with the kinda citrus-colored hair.
I'm going to cut straight to the chase and do a quick rundown of what we've learned about trigonometric identities recently. I got maybe three hours of sleep last night and I'm too lazy right now to include a bunch of pretty images and graphs, so have a billion colors instead.
Tanx is the trigonometric identity of sinx/cosx, therefore tanx = sinx/cosx. Anywhere you see tanx used in an equation, you can substitute sinx/cosx in for it, should that prove helpful in solving it. That's the general basis for a trigonometric identity.
Other standard trigonometric identities can be boiled down to the three seen on our formula sheet:
sin^2(x) + cos^2(x) = 1
tan^2(x) + 1 = sec^2(x)
1 + cot^2(x) = csc^2(x)
And a few derived by logic:
cscx = 1/sinx
secx = 1/cosx
cotx = 1/tanx
tanx = sinx/cosx
Ones such as:
a) cos^2(x) - sin^2(x) = 0,
Or even simplifying equations like these:
b) (cotx)/(cscx*cosx).
In order to solve a), we have to change that cos^2(x) into a sine function so we can simplify, or vice-versa. In order to do that, we can take the Pythagorean identity formula, and rearrange it to solve for cos^2(x).
After doing so, we will have something that looks like this: cos^2(x) = 1 - sin^2(x).
Now we can substitute 1 - sin^2(x) in for cos^2(x), and simplify the equation:
cos^2(x) - sin^2(x) = 0
1 - sin^2(x) - sin^2(x) = 0
-sin^2(x) - sin^2(x) + 1 = 0
sin^2(x) + sin^2(x) - 1 = 0
x^2 + x^2 - 1 = 0
2x^2 - 1 = 0
x^2 = 1/2
x = square-root(1/2)
sinx = square-root(1/2)
x reference = 45 degrees
The rest is history because I am lazy.
In order to solve b), we have to bring up something called:
It's simply a range of values an expression can't use for x, nothing too fancy. If you have an expression, 1/x, x obviously cannot equal 0, because dividing by zero yields an undefined value.
If we look at (cotx)/(cscx*cosx), we can see three trig functions here. A quick expansion/investigation of them shows us:
cotx = cosx/sinx
csc = 1/sinx
cosx = part of the product of a denominator
Judging by this, we can see that cosx =/= 0, and sinx =/= 0, because with a value of 0, we'd be dividing by 0 in more than one place, and we can't have that, no sir.
If we turn our attention towards the unit circle, the only places we see where cosx equaling 0 are at pi/2 + pi*n, where nEI.
If we also look again, we see the only places where sinx equals 0 are at pi*n, where nEI.
We can condense this information into a single equation to describe these non-permissible values.
x =/= (pi/2)*n, where nEI.
This equation must be coupled with the final simplified version of the equation, (cotx)/(cscx*cosx), for the final answer to be considered correct.
Now, in order to simplify this gross, ugly thing, we have to change all trig functions in the expression to either sin or cos functions, because this makes it nice and easy to handle. We've already partially done this while calculating the non-permissible values above, so we simply substitute the appropriate functions in for cot and csc.
(cotx)/(cscx*cosx)
(cosx/sinx)/((1/sinx)*cosx)
(cosx/sinx)/((1/sinx)*cosx/1)
(cosx/sinx)/(cosx/sinx)
= 1
As it turns out, that whole expression simplifies down to 1.
Toss the non-permissible values in there anyway, just for good measure.
(cotx)/(cscx*cosx) = 1
x =/= (pi/2)n, where nEI.
HHNGH I HAVEN'T EVEN GOTTEN TO DOUBLE ANGLE IDENTITIES YET.
Like this scary stuff:
a) sin48 * cos17 = cos48 * sin17
This even scarier stuff also:
b) sin(7pi/12) = x
Thankfully we get a ton of formulas on our formula sheets about this. Nearly the entire left side of the page is dedicated to this Alpha Beta nonsense.
I should probably list them here but there's a lot. Sorry people who don't have our formula sheet.
Pretend a=Alpha and B=Beta for the purposes of this blog post.
RIGHTO.
I have to sleep soon so I better get powering through this.
a)
(All angles are in degrees)
sin48 * cos17 - cos48 * sin17
Is the thing we got,
sina * cosB - cosa * sinB = sin(a - B)
Is the formula we can use.
Lining up the formulas like this, we can see that a should equal 48, and B should equal 17. We plug these values into our equation and do a quick calculation.
sin48 * cos17 - cos48 * sin17 = sin(48 - 17)
= sin(48 - 17)
= sin(31) is our expression written as a single trigonometric equation.
b)
sin(7pi/12) = x
This angle is clearly not one of our special triangles.
But you know what? We can make it out to be the sum of two special triangles.
What we do, is we take 7/12, omitting the pi for now, and break the 7 up into two values that add together to equal back to 7.
There are multiple sets of numbers like this:
2+5
4+3
1+6
But what we are looking for is a magical set of numbers that when put as the numerator, both divide cleanly by the denominator. Only 4+3 accomplishes this.
4/12 + 3/12
Simplify.
1/3 + 1/4
Now we reattach pi to the numerator.
pi/3 + pi/4
We now see how sin(7pi/12) = sin(pi/3 + pi/4)
We can use these two new angles as our Alpha and Beta respectively. We select the appropriate formula: sin(a + B) = sina * cosB + cosa * sinB and plug these new values in:
sin(pi/3) * cos(pi/4) + cos(pi/3) * sin(pi/4)
Now, according to the special triangles associated with each angle, we can pick out the exact values of each trig function.
[sqroot(3)/2 * 1/sqroot(2)] + [1/2 * 1/sqroot(2)]
Simplify...
[sqroot(3)/2sqroot(2)] + [1/2sqroot(2)]
Simplify again...
[sqroot(3)+1]/[2sqroot(2)]
This is the exact value of sin(7pi/12)
Oops haha now I have no time left for the rest of my homework.
Hope this helped someone. I'm proud of my big messy colored number explosion.
Hope I didn't make any stupid errors like I keep doing now for some reason.
After wrestling with Blogger for an hour I finally figured out where my invitation email went. So uh, hi guys, I don't want to do this but I'm being forced, I guess. This is Casey, that quiet girl in the back of the classroom with the kinda citrus-colored hair.
I'm going to cut straight to the chase and do a quick rundown of what we've learned about trigonometric identities recently. I got maybe three hours of sleep last night and I'm too lazy right now to include a bunch of pretty images and graphs, so have a billion colors instead.
What is a trigonometric identity exactly?
A trigonometric identity is essentially a couple of trig functions in disguise.Tanx is the trigonometric identity of sinx/cosx, therefore tanx = sinx/cosx. Anywhere you see tanx used in an equation, you can substitute sinx/cosx in for it, should that prove helpful in solving it. That's the general basis for a trigonometric identity.
Other standard trigonometric identities can be boiled down to the three seen on our formula sheet:
sin^2(x) + cos^2(x) = 1
tan^2(x) + 1 = sec^2(x)
1 + cot^2(x) = csc^2(x)
And a few derived by logic:
cscx = 1/sinx
secx = 1/cosx
cotx = 1/tanx
tanx = sinx/cosx
How can I use trigonometric identities?
During this course, you will have to solve a multitude of daunting looking trigonometric equations.Ones such as:
a) cos^2(x) - sin^2(x) = 0,
Or even simplifying equations like these:
b) (cotx)/(cscx*cosx).
In order to solve a), we have to change that cos^2(x) into a sine function so we can simplify, or vice-versa. In order to do that, we can take the Pythagorean identity formula, and rearrange it to solve for cos^2(x).
After doing so, we will have something that looks like this: cos^2(x) = 1 - sin^2(x).
Now we can substitute 1 - sin^2(x) in for cos^2(x), and simplify the equation:
cos^2(x) - sin^2(x) = 0
1 - sin^2(x) - sin^2(x) = 0
-sin^2(x) - sin^2(x) + 1 = 0
sin^2(x) + sin^2(x) - 1 = 0
x^2 + x^2 - 1 = 0
2x^2 - 1 = 0
x^2 = 1/2
x = square-root(1/2)
sinx = square-root(1/2)
x reference = 45 degrees
The rest is history because I am lazy.
In order to solve b), we have to bring up something called:
Non-permissible values
Oh gosh what is all this nonsense now?It's simply a range of values an expression can't use for x, nothing too fancy. If you have an expression, 1/x, x obviously cannot equal 0, because dividing by zero yields an undefined value.
If we look at (cotx)/(cscx*cosx), we can see three trig functions here. A quick expansion/investigation of them shows us:
cotx = cosx/sinx
csc = 1/sinx
cosx = part of the product of a denominator
Judging by this, we can see that cosx =/= 0, and sinx =/= 0, because with a value of 0, we'd be dividing by 0 in more than one place, and we can't have that, no sir.
If we turn our attention towards the unit circle, the only places we see where cosx equaling 0 are at pi/2 + pi*n, where nEI.
If we also look again, we see the only places where sinx equals 0 are at pi*n, where nEI.
We can condense this information into a single equation to describe these non-permissible values.
x =/= (pi/2)*n, where nEI.
This equation must be coupled with the final simplified version of the equation, (cotx)/(cscx*cosx), for the final answer to be considered correct.
Now, in order to simplify this gross, ugly thing, we have to change all trig functions in the expression to either sin or cos functions, because this makes it nice and easy to handle. We've already partially done this while calculating the non-permissible values above, so we simply substitute the appropriate functions in for cot and csc.
(cotx)/(cscx*cosx)
(cosx/sinx)/((1/sinx)*cosx)
(cosx/sinx)/((1/sinx)*cosx/1)
(cosx/sinx)/(cosx/sinx)
= 1
As it turns out, that whole expression simplifies down to 1.
Toss the non-permissible values in there anyway, just for good measure.
(cotx)/(cscx*cosx) = 1
x =/= (pi/2)n, where nEI.
HHNGH I HAVEN'T EVEN GOTTEN TO DOUBLE ANGLE IDENTITIES YET.
Using double angle identities
Double angle identities are pretty much the exact same thing as above, except used for, but not limited to, when we have stuff to solve or prove or calculate that involves more than one angle crammed into an equation.Like this scary stuff:
a) sin48 * cos17 = cos48 * sin17
This even scarier stuff also:
b) sin(7pi/12) = x
Thankfully we get a ton of formulas on our formula sheets about this. Nearly the entire left side of the page is dedicated to this Alpha Beta nonsense.
I should probably list them here but there's a lot. Sorry people who don't have our formula sheet.
Pretend a=Alpha and B=Beta for the purposes of this blog post.
RIGHTO.
I have to sleep soon so I better get powering through this.
a)
(All angles are in degrees)
sin48 * cos17 - cos48 * sin17
Is the thing we got,
sina * cosB - cosa * sinB = sin(a - B)
Is the formula we can use.
Lining up the formulas like this, we can see that a should equal 48, and B should equal 17. We plug these values into our equation and do a quick calculation.
sin48 * cos17 - cos48 * sin17 = sin(48 - 17)
= sin(48 - 17)
= sin(31) is our expression written as a single trigonometric equation.
b)
sin(7pi/12) = x
This angle is clearly not one of our special triangles.
But you know what? We can make it out to be the sum of two special triangles.
What we do, is we take 7/12, omitting the pi for now, and break the 7 up into two values that add together to equal back to 7.
There are multiple sets of numbers like this:
2+5
4+3
1+6
But what we are looking for is a magical set of numbers that when put as the numerator, both divide cleanly by the denominator. Only 4+3 accomplishes this.
4/12 + 3/12
Simplify.
1/3 + 1/4
Now we reattach pi to the numerator.
pi/3 + pi/4
We now see how sin(7pi/12) = sin(pi/3 + pi/4)
We can use these two new angles as our Alpha and Beta respectively. We select the appropriate formula: sin(a + B) = sina * cosB + cosa * sinB and plug these new values in:
sin(pi/3) * cos(pi/4) + cos(pi/3) * sin(pi/4)
Now, according to the special triangles associated with each angle, we can pick out the exact values of each trig function.
[sqroot(3)/2 * 1/sqroot(2)] + [1/2 * 1/sqroot(2)]
Simplify...
[sqroot(3)/2sqroot(2)] + [1/2sqroot(2)]
Simplify again...
[sqroot(3)+1]/[2sqroot(2)]
This is the exact value of sin(7pi/12)
Oops haha now I have no time left for the rest of my homework.
Hope this helped someone. I'm proud of my big messy colored number explosion.
Hope I didn't make any stupid errors like I keep doing now for some reason.
Thursday, November 21, 2013
Hi there! My name is Sanvir most of u know me.Hopefully you all had a good circular functions test not like me so I am here to review a little bit of concept as I found on the internet which might be helpful to you.
Things we should know;
-converting radians to degrees, degrees to radians
- Arc length questions,
- finding standard position and finding reference angles
- solve for theta given different ranges
- exact values
- stretchs/ compressions, vertical/ horizontal shifts in functions
- unit circle
and so on.
Things we should know;
-converting radians to degrees, degrees to radians
- Arc length questions,
- finding standard position and finding reference angles
- solve for theta given different ranges
- exact values
- stretchs/ compressions, vertical/ horizontal shifts in functions
- unit circle
and so on.
As you all know CAST rule is one of the important things we need to remember so I updated one of the picture representing it
Moving forward I found some important questions related to trigonometric graphs that might also help you.Sorry i don't write too much as my English is too bad.
And if u didn't got that calculator question right then this video will help.In these video there are four different type of examples shown to solve trig equations
Have a nice weekend and if didn't find my information helpful I am sorry about that and will try better next time !
Saturday, November 2, 2013
Special Angles, Multiples, and Patterns
Hi there! My name is Patricia Uy (a.k.a Pat ). For those who don't know me, I am the short, talkative girl who sits at the back of the classroom (the one with the bangs). I will be summarizing the lessons from yesterday's Pre-Calculus 40S classes. I originally planned on doing this yesterday after school, but I kinda fell asleep....anyway, let's get started with the math!
As you can see, the sinθ and cosθ values for the angles and their multiples repeat after crossing the x or y axis (they just differ in sign). This is due to their location on a coordinate plane (quadrant).
Next, we looked at the Unit Circle sheet. It has all of the degrees, multiples, exact radians, and the coordinates for sin and cos.
To solve these questions, you must first understand the concept of reference angles, special triangles, co terminal angles, SOH CAH TOA, and the CAST rule. If you are unsure about these, I strongly suggest checking out my friend, Jasiel Rosario's post that explains the basics of Special Right Triangles.
a) P(13π/2)=(x, y)
Just by looking at the given value, we can already see that our reference angle is π/2.
If π =180°, that must mean that π/2 = 90°. Looking back at the Unit Circle sheet, π/2 falls on the y-axis with the coordinates (0,1). Therefore, P(13π/2) also has the same coordinates. If you are confused as to why this is, I suggest counting counter-clockwise by 90°s (1/4) around the circle for 13 times. You will end up on the y-axis.
Answer:
P(13π/2)=(x, y)
P(13π/2)=(0,1)
b) P(-9π/4)=(x,y)
Our reference angle this time will be π/4. We can use this information to figure out what type of special triangle we are dealing with.
If π =180°, π/4= 45°. Our special triangle is:
We learned during our previous classes that (x, y)=(cosθ, sinθ). With this in mind, we can start solving for the coordinates!
First, we need to find cos and sin. According to SOH CAH TOA, cos = adjacent/hypotenuse and sin =opposite/hypotenuse.
In this case, cos = 1/√2 and sin = 1/√2.
Then you plug these into (cosθ, sinθ)! You will end up with (1/√2, 1/√2).
The final step is to apply the CAST rule. This will help you determine the signs of your coordinates. If you were to count clockwise (because negative) around the circle by 45°s (1/8) for 9 times, you will find yourself in Quadrant 4. Quadrant 4 is the "C" section, meaning everything else but cos must be negative. After applying this to the coordinates we obtained earlier, the answer should be (1/√2, -1/√2).
Answer:
P(-9π/4)=(x,y)
P(-9π/4)=(1/√2, -1/√2)
You will need to follow the same steps in order to solve c) and d).
During our afternoon class, we learned how to solve for the measurement of central angle θ when given an interval.
This is basically like the previous questions we solved but reversed. Remember, the given interval is 0≤θ≤2π.
a) P(θ)=(0,1)
Based on the question we solved earlier, we know that the coordinates for π/2 is (0,1) because it lies on the y-axis. Therefore, the the measurement of θ should be π/2.
Answer:
P(θ)=(0,1)
P(θ)=π/2
b) P(θ)=(-1/√2, -√3/2)
The given cos and sin value are negative. According to the CAST rule, the only quadrant where cos and sin are both negative is quadrant 3.
Because the cos value is (-1/√2) and the sin value is (-√3/2), our special triangle must be:
We can now start labeling our special triangle.
Opp= -√3, adj= -1, hyp= 2 (hypotenuse will always be positive). The degree is 60.
Now that we know what the degree is, we can find the reference angle. The reference angle for this question is π/3. All we have to do now is to count counter-clockwise by 60°s (1/6) around the circle until we reach our triangle. It should take us 4 times. Therefore, our θ should be 4π/3.
Answer:
P(θ)=(-1/√2, -√3/2)
θ= 4π/3
You will need to follow these steps in order to solve c) and d).
Mr. P then proceeded into teaching us how to find the coordinates of P(θ) when it is rotated.
There are certain patterns we can use when determining the coordinates of points on the unit circle!
Pattern #1: When asked to rotate the original θ to a half rotation (π), P(θ ± π) will result in the same x and y values but their signs will depend on which quadrant they are on.
Example 2:
P(θ ± π)=(3/5, -4/5), find exact coordinates of P(θ+π) and P(θ-π).
Based on the coordinates given:
cosθ=adj/hyp
cosθ=3/5
sinθ=opp/hyp
sinθ=-4/5
If we plot this point on our circle, it would be on quadrant 4. This is because our cos value is positive and sin value is negative (CAST rule).
Now if we rotated it by half or π(direction does not matter in this case because half rotations in either direction will result in the same point), we will end up in quadrant 2.
We then need to apply the CAST rule. Because we are now in quadrant 2, our cos must be negative and our sin must be positive. The point will then have the coordinates (-3/5, 4/5).
Answer:
P(θ ± π)=(-3/5, 4/5)
Pattern #2: When asked to rotate original θ to quarter rotation, P(θ ± π/2) will result in switched x and y values. Just like the previous pattern, the signs depend on the quadrant.
Example 2:
P(θ)=(3/5, -4/5), find the exact coordinates of P(θ+π/2) and P(θ-π/2).
Based on the given coordinates:
cosθ=adj/hyp
cosθ=3/5
sinθ=opp/hyp
sinθ=-4/5
If we plot this point on our circle, it would be on quadrant 4.
Now if we rotate it clockwise by a quarter(π/2), we will end up in quadrant 3. If we rotate it counter-clockwise, we will end up in quadrant 1 (direction in this case matters because both ways will result in different points).
The point on quadrant 3 will have the coordinates (-4/5, -3/5). The values are switched and both must be negative (CAST rule).
The point on quadrant 1 will have the coordinates (4/5, 3/5). The values are switched and both must be positive (CAST rule).
Answer:
P(θ-π/2)=(-4/5, -3/5)
P(θ+π/2)=(4/5, 3/5)
Well, that's about it! I hope my explanations were clear and helpful :) If you are still having trouble understanding these lessons, here's a video that might help you: http://www.youtube.com/watch?v=hzucE6S5SXM. Have a great weekend and see y'all on Monday!!!
<3 Pat
P.S. I was browsing through the Internet yesterday (yes, before I fell asleep) and BAM! I saw this:
During our morning class, Mr. P first went over the Special Angles and Multiples and Fill in The Unit Circle sheets that he assigned for homework the day before. In case you missed it, here they are:
(I would like to apologize in advance for the tiny notes/scribbles on the sides of the sheets. Please ignore them!)
As you can see, the sinθ and cosθ values for the angles and their multiples repeat after crossing the x or y axis (they just differ in sign). This is due to their location on a coordinate plane (quadrant).
30°-90°= Quadrant 1
120°-180°= Quadrant 2
210°-270°= Quadrant 3
300°-360°= Quadrant 4
This is all in reference to the CAST RULE.
Next, we looked at the Unit Circle sheet. It has all of the degrees, multiples, exact radians, and the coordinates for sin and cos.
After that, the class proceeded into solving for the exact coordinates of P(θ).
To solve these questions, you must first understand the concept of reference angles, special triangles, co terminal angles, SOH CAH TOA, and the CAST rule. If you are unsure about these, I strongly suggest checking out my friend, Jasiel Rosario's post that explains the basics of Special Right Triangles.
a) P(13π/2)=(x, y)
Just by looking at the given value, we can already see that our reference angle is π/2.
If π =180°, that must mean that π/2 = 90°. Looking back at the Unit Circle sheet, π/2 falls on the y-axis with the coordinates (0,1). Therefore, P(13π/2) also has the same coordinates. If you are confused as to why this is, I suggest counting counter-clockwise by 90°s (1/4) around the circle for 13 times. You will end up on the y-axis.
Answer:
P(13π/2)=(x, y)
P(13π/2)=(0,1)
b) P(-9π/4)=(x,y)
Our reference angle this time will be π/4. We can use this information to figure out what type of special triangle we are dealing with.
If π =180°, π/4= 45°. Our special triangle is:
 |
| Opp=1, Adj=1, Hyp=√2 |
We learned during our previous classes that (x, y)=(cosθ, sinθ). With this in mind, we can start solving for the coordinates!
First, we need to find cos and sin. According to SOH CAH TOA, cos = adjacent/hypotenuse and sin =opposite/hypotenuse.
In this case, cos = 1/√2 and sin = 1/√2.
Then you plug these into (cosθ, sinθ)! You will end up with (1/√2, 1/√2).
The final step is to apply the CAST rule. This will help you determine the signs of your coordinates. If you were to count clockwise (because negative) around the circle by 45°s (1/8) for 9 times, you will find yourself in Quadrant 4. Quadrant 4 is the "C" section, meaning everything else but cos must be negative. After applying this to the coordinates we obtained earlier, the answer should be (1/√2, -1/√2).
Answer:
P(-9π/4)=(x,y)
P(-9π/4)=(1/√2, -1/√2)
You will need to follow the same steps in order to solve c) and d).
During our afternoon class, we learned how to solve for the measurement of central angle θ when given an interval.
This is basically like the previous questions we solved but reversed. Remember, the given interval is 0≤θ≤2π.
a) P(θ)=(0,1)
Based on the question we solved earlier, we know that the coordinates for π/2 is (0,1) because it lies on the y-axis. Therefore, the the measurement of θ should be π/2.
Answer:
P(θ)=(0,1)
P(θ)=π/2
b) P(θ)=(-1/√2, -√3/2)
The given cos and sin value are negative. According to the CAST rule, the only quadrant where cos and sin are both negative is quadrant 3.
Because the cos value is (-1/√2) and the sin value is (-√3/2), our special triangle must be:
 |
| Opp=√3, Adj=1, Hyp=2 |
We can now start labeling our special triangle.
Opp= -√3, adj= -1, hyp= 2 (hypotenuse will always be positive). The degree is 60.
Now that we know what the degree is, we can find the reference angle. The reference angle for this question is π/3. All we have to do now is to count counter-clockwise by 60°s (1/6) around the circle until we reach our triangle. It should take us 4 times. Therefore, our θ should be 4π/3.
Answer:
P(θ)=(-1/√2, -√3/2)
θ= 4π/3
You will need to follow these steps in order to solve c) and d).
Mr. P then proceeded into teaching us how to find the coordinates of P(θ) when it is rotated.
There are certain patterns we can use when determining the coordinates of points on the unit circle!
Pattern #1: When asked to rotate the original θ to a half rotation (π), P(θ ± π) will result in the same x and y values but their signs will depend on which quadrant they are on.
Example 2:
P(θ ± π)=(3/5, -4/5), find exact coordinates of P(θ+π) and P(θ-π).
Based on the coordinates given:
cosθ=adj/hyp
cosθ=3/5
sinθ=opp/hyp
sinθ=-4/5
If we plot this point on our circle, it would be on quadrant 4. This is because our cos value is positive and sin value is negative (CAST rule).
Now if we rotated it by half or π(direction does not matter in this case because half rotations in either direction will result in the same point), we will end up in quadrant 2.
We then need to apply the CAST rule. Because we are now in quadrant 2, our cos must be negative and our sin must be positive. The point will then have the coordinates (-3/5, 4/5).
Answer:
P(θ ± π)=(-3/5, 4/5)
Pattern #2: When asked to rotate original θ to quarter rotation, P(θ ± π/2) will result in switched x and y values. Just like the previous pattern, the signs depend on the quadrant.
Example 2:
P(θ)=(3/5, -4/5), find the exact coordinates of P(θ+π/2) and P(θ-π/2).
Based on the given coordinates:
cosθ=adj/hyp
cosθ=3/5
sinθ=opp/hyp
sinθ=-4/5
If we plot this point on our circle, it would be on quadrant 4.
Now if we rotate it clockwise by a quarter(π/2), we will end up in quadrant 3. If we rotate it counter-clockwise, we will end up in quadrant 1 (direction in this case matters because both ways will result in different points).
The point on quadrant 3 will have the coordinates (-4/5, -3/5). The values are switched and both must be negative (CAST rule).
The point on quadrant 1 will have the coordinates (4/5, 3/5). The values are switched and both must be positive (CAST rule).
Answer:
P(θ-π/2)=(-4/5, -3/5)
P(θ+π/2)=(4/5, 3/5)
Well, that's about it! I hope my explanations were clear and helpful :) If you are still having trouble understanding these lessons, here's a video that might help you: http://www.youtube.com/watch?v=hzucE6S5SXM. Have a great weekend and see y'all on Monday!!!
<3 Pat
P.S. I was browsing through the Internet yesterday (yes, before I fell asleep) and BAM! I saw this:
 |
| As I am writing this, my mom is staring at me with a WTH face because I'm laughing like a maniac. Thank you Clay Aiken. |
Friday, November 1, 2013
A Random, Insightful Announcement
Hey guys! It's Charina, again. I'm not here to scribe, talk about "tall, handsome" men, or promote another secret blog. In fact, I'm not going to talk about Pre-Calculus at all. I know that you are all studying hard on all of your courses this term, but if you have time please drop by the Central Canada Comic Con at the Winnipeg Convention Centre!
First of all, what is Comic Con? Well, if you like comic books (Legion, Daredevil, Batman, Superman, etc.), video games (Assassin's Creed, Uncharted, Portal, etc.), Science Fiction, and stuff like that -- this place is for you. But if it's not your thing, that's alright too! We don't exclusively accommodate to comic-book/video game lovers, we have vendors who sell anime, Asian imports (*cough* Green tea Kit Kat *cough*), art supplies, crafts, posters, novelty items -- all of that fun stuff. The con is officially up and running today, tomorrow and Sunday. Tickets can be purchased through Ticketmaster, which is also at the Convention Centre:
Weekend Passes - $37.00
Child Weekend Passes - $22.00
Adult Day Pass - $17.00
Child Day Pass - $17.00
A man taking the census walks up to the apartment of a mathematician and asks him if he has any children and how old they are. The mathematician says "I have three daughters and the product of their ages is 72." The man tells the mathematician that he needs more information, so the mathematician tells him "The sum of their ages is equal to our apartment number." The man still needs more information so the mathematician tells him "My oldest daughter has her own bed and the other two share bunk beds."
How old are his daughters?
18, 2 and 2.
9, 4 and 2.
6, 6 and 2.
6, 4 and 3.
8, 3, and 3.
Since he doesn't know the ages after this piece of information the sum of the three numbers must not be unique. The sum of 8, 3, and 3; and 6, 6, and 2 are the same. Now the final clue comes in handy. Since we know that the oldest daughter has her own bed it is likely that she has the bed to herself and is older than the other two so there ages are 8, 3, and 3 rather than 2, 6 and 6.
Hey Everyone!! I am "student #23" a.k.a, Jasiel Rosario and it is finally my turn to share to our class blog about what we learned today in Pre-Calculus 40S. Most of you probably do not know me because I am just a new student here at Maples, and I just migrated here to Canada 3 months ago. If you want to see me, well, I am just the third person next to Mr.P's desk, and I sit next to Jamie and Nick. Okay, enough with all the introduction, let's start doing MATH. Yippiiiieeee!
Our topic for today is Special Right Triangles. Using these triangles, we can be able to determine the exact values of trig ratios for any multiples of 0˚, 30˚ 45˚ and 60˚.There are three points for this topic:
I. Six Trigonometric Functions- To be able to understand this unit, we should be familiar with the six trigonometric functions and memorize them by heart. The primary functions are sine, cosine, and tangent.
30º- 60º- 90º triangle
By using a triangle with a side of 2 units, you can determine the ratios in a triangle with angles of
30˚, 60˚, and 90˚.
If we drop a vertical line that bisects the base we get two right triangles with these angles.

If a=1 and c=2
b=?
To solve for b, once again we use the Pythagorean Theorem.
b2 = c2 - a2
b2 = (2)2 - (1)2
b= √3
Once again, we use the SOHCAHTOA to get the exact values of the trigonometric functions.
* Quadrantal Angles- These are angles whose terminal side lies on the x or y-axis. The vales of their functions or coordinates, are also exact. They do not have decimal values.

Our topic for today is Special Right Triangles. Using these triangles, we can be able to determine the exact values of trig ratios for any multiples of 0˚, 30˚ 45˚ and 60˚.There are three points for this topic:
I. Six Trigonometric Functions- To be able to understand this unit, we should be familiar with the six trigonometric functions and memorize them by heart. The primary functions are sine, cosine, and tangent.
a= adjacent side
o=opposite side
h=hypotenuse
The three remaining functions can actually be derived from the primary functions. They are also called, "Reciprocal Functions" because their values corrrespond to the reciprocal of the primary functions.
II. Special Angles- most angles on the unit circle are based on reference angles of either 30˚, 45˚, and 60˚.
III. Reference Triangles- Using the nature of these triangles will help us determine the exact values of the trigonometric functions. These are the 45º-
45º- 90º triangle and the 30º-
60º- 90º triangle.
45º-
45º- 90º Triangle
In the figure above, the 2 legs (a and b) are congruent.
Let a=1
b=1
c= ?
Now, using the Pythagorean Theorem we should be able to determine the value of our hypotenuse.
a2 + b2 = c
(1)2 + (1)2 = c
c=√2
Since we now know the value of our hypotenuse, we use the SOHCAHTOA formula to tell the EXACT values of our trig functions for any multiple of 45˚,
or π/4.
By using a triangle with a side of 2 units, you can determine the ratios in a triangle with angles of
30˚, 60˚, and 90˚.
If we drop a vertical line that bisects the base we get two right triangles with these angles.

If a=1 and c=2
b=?
To solve for b, once again we use the Pythagorean Theorem.
b2 = c2 - a2
b2 = (2)2 - (1)2
b= √3
Once again, we use the SOHCAHTOA to get the exact values of the trigonometric functions.
(Here is a sample of a graph of a circular function. Based on the coordinates of the quadrantal angles, we were able to plot out the points of the function. The graph of a circular function will be discussed further in the future lessons.)
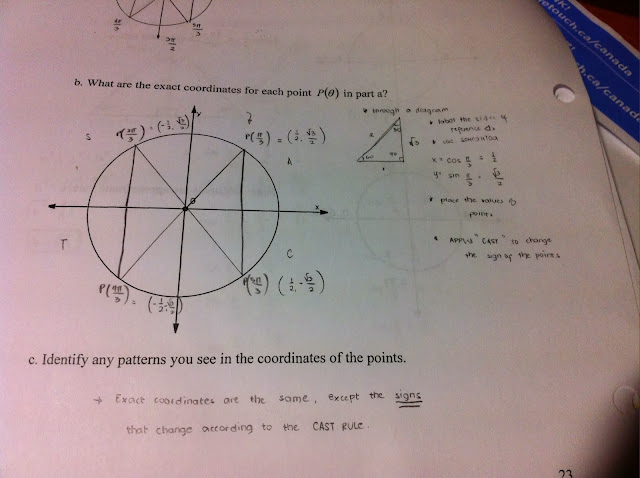
To get the coordinates for any multiples of our special angles, Mr.P taught us how to do these examples:
Example 1: Multiples of π/3
First of all, we get the integral multiples.
Second, we get the EXACT coordinates.
Use the SOHCAHTOA formula once again to get the value of the x-coordinate (cosine) and the y-coordinate(sine).
Finally, apply the "CAST" method to change the signs of the coordinates.
Now apply the same method to get the exact coordinates for the multiples of π/6 and π/4. Once you have done that, voila! You have uncovered all the missing pieces of the puzzle and you can now complete the unit circle.
Okay! That covers everything that we have discussed today about circular functions. I hope I explained everything clearly and this will be helpful to you. :)
Subscribe to:
Posts (Atom)








-page-001.JPG)