Hey Everyone!! I am "student #23" a.k.a, Jasiel Rosario and it is finally my turn to share to our class blog about what we learned today in Pre-Calculus 40S. Most of you probably do not know me because I am just a new student here at Maples, and I just migrated here to Canada 3 months ago. If you want to see me, well, I am just the third person next to Mr.P's desk, and I sit next to Jamie and Nick. Okay, enough with all the introduction, let's start doing MATH. Yippiiiieeee!

Our topic for today is Special Right Triangles. Using these triangles, we can be able to determine the exact values of trig ratios for any multiples of 0˚, 30˚ 45˚ and 60˚.There are three points for this topic:

I. Six Trigonometric Functions- To be able to understand this unit, we should be familiar with the six trigonometric functions and memorize them by heart. The primary functions are sine, cosine, and tangent.

 

a= adjacent side

o=opposite side

h=hypotenuse

The three remaining functions can actually be derived from the primary functions. They are also called, "Reciprocal Functions" because their values corrrespond to the reciprocal of the primary functions.

II. Special Angles- most angles on the unit circle are based on reference angles of either 30˚, 45˚, and 60˚.

III. Reference Triangles- Using the nature of these triangles will help us determine the exact values of the trigonometric functions. These are the 45º- 45º- 90º triangle and the 30º- 60º- 90º triangle.

 45º- 45º- 90º Triangle

 In the figure above, the 2 legs (a and b) are congruent.

Let a=1

      b=1

      c= ?

Now, using the Pythagorean Theorem we should be able to determine the value of our hypotenuse.

a² + b² = c

(1)² + (1)² = c

c=√2

 Since we now know the value of our hypotenuse, we use the SOHCAHTOA formula to tell the EXACT values of our trig functions for any multiple of 45˚, or π/4.

30º- 60º- 90º triangle 

By using a triangle with a side of 2 units, you can determine the ratios in a triangle with angles of

30˚, 60˚, and 90˚. 

 If we drop a vertical line that bisects the base we get two right triangles with these angles.

If a=1 and c=2
b=?




 To solve for b, once again we use the Pythagorean Theorem.

  b² = c^{2  -} a²   
    b² = (2)^{2  -} (1)<sup>2

   b= √3

Once again, we use the SOHCAHTOA to get the exact values of the trigonometric functions.</sup>

  
   

 * Quadrantal Angles- These are angles whose terminal side lies on the x or y-axis. The vales of their functions or coordinates, are also exact. They do not have decimal values. 

(Here is a sample of a graph of a circular function. Based on the coordinates of the quadrantal angles, we were able to plot out the points of the function. The graph of a circular function will be discussed further in the future lessons.)

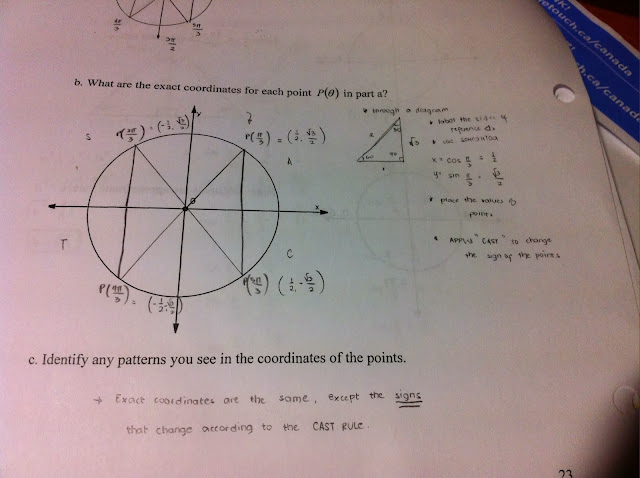
To get the coordinates for any multiples of our special angles, Mr.P taught us how to do these examples:

Example 1: Multiples of π/3 

First of all, we get the integral multiples. 

Second, we get the EXACT coordinates.

Use the SOHCAHTOA formula once again to get the value of the x-coordinate (cosine) and the y-coordinate(sine). 

Finally, apply the "CAST" method to change the signs of the coordinates.

Now apply the same method to get the exact coordinates for the multiples of π/6 and π/4. Once you have done that, voila! You have uncovered all the missing pieces of the puzzle and you can now complete the unit circle. 

Okay! That covers everything that we have discussed today about circular functions. I hope I explained everything clearly and this will be helpful to you. :)